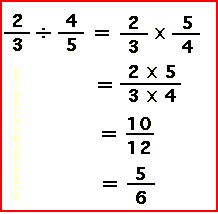Check out these website for fun activies for more practice:
Kids Math
COOL GAME 4 Kids
FUN&BRAIN
MATH GAMES
COOL MATHS
Tuesday, June 2, 2009
BONUS QUESTIONS?

Give these questions a try:
—1. Jessica bought 8/9 of a pound of chocolates and ate1/3 of a pound. How much was left?
—2. Sally walked 3/4 of a mile before lunch and 1/2 of a mile after lunch. How far did she walk in all?
—3. Don bought 3/4 of a pound of jellybeans and 5/8 pound of gummy bears. How much candy did he buy?
—4.Which apple weighs more, one that weighs 2/3 of a pound or one that weighs 5/6 of a pound?
—5. Stanley ordered two pizzas cut into eighths. If he ate 5/8 of a pizza, how much was left?
HOMEWORK --- Fractions: Multiplication & Division
—Multiplication rules:
1.Multiply 1st numerator to 2nd numerator.
2.Multiply 1st denominator to 2nd denominator.
Example:
—Division rules:
1.Keep the first fraction.
2.Change sign.
3.Switch number for the 2nd fraction
4.Then multiply
Example:
Homework section: READ, PRINT, SHOW WORK, AND TURN THEM IN ON TIME :<)
Rules for HOMEWORK --- MUST READ!!!!!!
In this blog, students will have 3 homeworks to finish and turn in. You must read the instructions to do the homework:
1. Read the notes concerning about fractions.
2. Call and email me if you have questions related to the homework.
3. Print out the pages, or ask me for the copy if you can' print them.
4. Write out the answer on a blank paper with the answer and the problem and show your work.
5. ALL homework must turn in on the due date or will get grade deduction.
6. Make sure your name, date, topic, teacher's name are on the top of the left corner when turn in the homework.
When you're in trouble ask question; ask me or your friends.
Email or call me whenever you need. I'll answer them right away :)
One more thing, there is a bonus section with some problems that you can answer or just try them out. I will give you 100% for try out the problem; I will give you 200 % for have them all correct. Anyhow, just some problems; give it a try and if you get stuck on something ask me and I will give you some hints.
Welcome Students

Welcome to my page...
Read the following rules before browsing the blog:
Read the following rules before browsing the blog:
1. You can check out the homework section, the notes sections, and you can post questions or comments. *I will answer all questions, as soon as I see them
2. You can search for more information about math and upload them with my permission :)
3. Every student must uses adequate language, no profanity and no words like: "That's stupid," "Shut up," etc...
4. You must follow the rules in order to allow on to the blog.
5. Check out all the links to play games and to practice problems before try out the homework.
6. Students who do not follow the rules will get points taken away and no free time on the computer.
7. Students will get punishment in class for disrupt or break any of the blogs rules.
8. Homework section - read direction before attempt to work on them ( 3 worksheets must turn in )
9. Bonus section - optional, but you will get 100% for trying.
Well, check out the blog and have fun...
All About FRACTION

Fractions were invented long before decimal numbers, as a way of showing portions less than 1, and they're still hanging around.
They're used in cooking, in building, in sewing, in the stock market - they're everywhere, and we need to understand them.
3 (numerator)
__4 (denominator) or written as 3/4
We can read this fraction as three-fourths, three over four, or three divided by four.
3/4 = 0.75
Every fraction can be converted to a decimal by dividing. If you use the calculator to divide 3 by 4, you'll find that it is equal to 0.75.
Every fraction can be converted to a decimal by dividing. If you use the calculator to divide 3 by 4, you'll find that it is equal to 0.75.
More examples:
1/2 = 0.5
1/4 = 0.25
1/8 = 0.125
Here are some terms that are very important when working with fractions.
Proper fraction: When the numerator is less than the denominator, we call the expression a proper fraction. These are some examples of proper fractions:
3/4
2/3
1/9
97/100
Improper fraction: An improper fraction occurs when the numerator is greater than or equal to the denominator. These are some examples of improper fractions:
5/4
9/2
6/6
19/7
Mixed number: When an expression consists of a whole number and a proper fraction, we call it a mixed number. Here are some examples of mixed numbers:

We can convert a mixed number to an improper fraction. First, multiply the whole number by the denominator of the fraction. Then, add the numerator of the fraction to the product. Finally, write the sum over the original denominator.
In this example, since three thirds is a whole, the whole number 1 is three thirds plus one more third, which equals four thirds.
Convert 1-1/3 to an improper fraction:
(1x3)+1 4
---------=---
3 3
Equivalent fractions: There are many ways to write a fraction of a whole. Fractions that represent the same number are called equivalent fractions. This is basically the same thing as equal ratios. For example: 2/4, and 4/8 are all equivalent fractions. To find out if two fractions are equivalent, use a calculator and divide. If the answer is the same, then they are equivalent.
Subscribe to:
Comments (Atom)